Sample Size Formula For Quantitative Research

Determining the sample size in a quantitative research study is challenging.

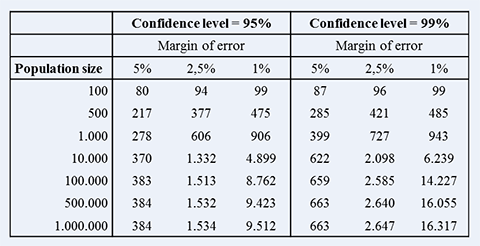
Sample size formula for quantitative research. Your sample size is the amount of consumers in your target population that you will be researching. The minimum amount of consumers you need to research for your results to be statistically significant within your defined parameters. However they may be of little value in early exploratory studies where scarce data are available on which to base the calculations though this may be addressed by performing a pilot study first and using the data from that. Sample size calculation is important to understand the concept of the appropriate sample size because it is used for the validity of research findings.

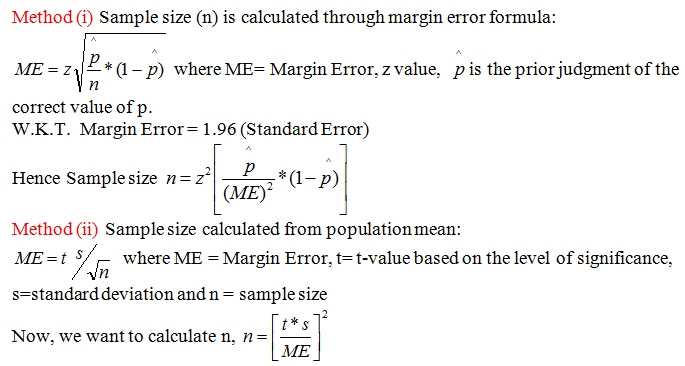
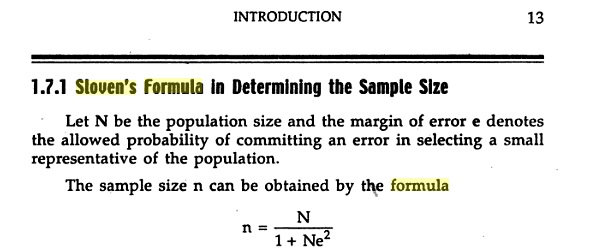
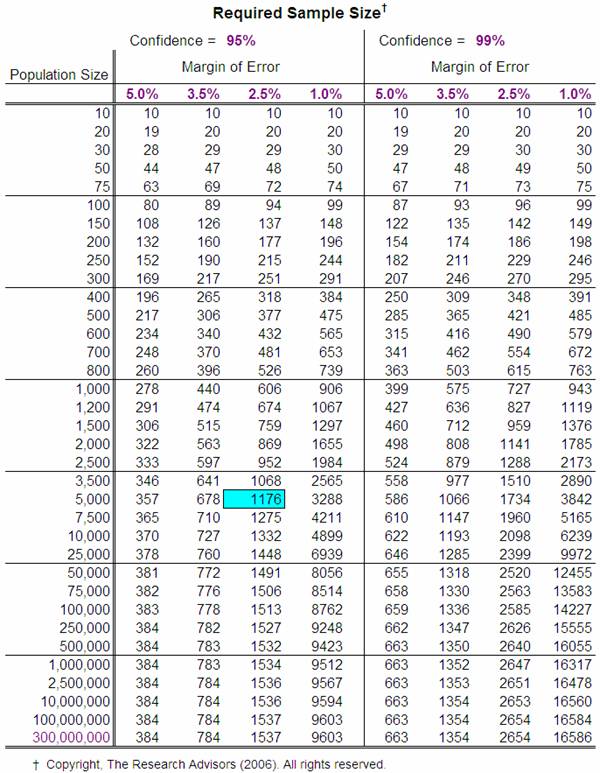
Sample is the part of the population that helps us to draw inferences about the population. In case it is too small it will not yield valid results while a sample is too large may be a waste of both money and time. Thus we need an appropriate sample size so that we can make inferences about the population based on that sample. Krejcie and morgan s 1970 formula for determining sample size for categorical data will be briefly discus sed because it provides identical sample sizes in all cases where the researcher adjusts the t value used based on.

In this research method researchers and statisticians deploy mathematical frameworks and theories that pertain to the quantity under question. Recall that typical quantitative research seeks to infer from a sample to a population for example a relationship or a treatment effect. Typically there are three factors or variables one must know about a given study each. The stages of quantitative research the stage in which we are sampling the importance of sampling for a research deciding on sample size and sampling methods are summarised briefly.

Quantitative outcome research is mostly conducted in the social sciences using the statistical methods used above to collect quantitative data from the research study. Each experiment is different with varying degrees of certainty and expectation. The appropriate use of cochran s 1977 sample size formula for both continuous and categorical data will be presented. Collecting research of the complete information about the population is not possible and it is time consuming and expensive.

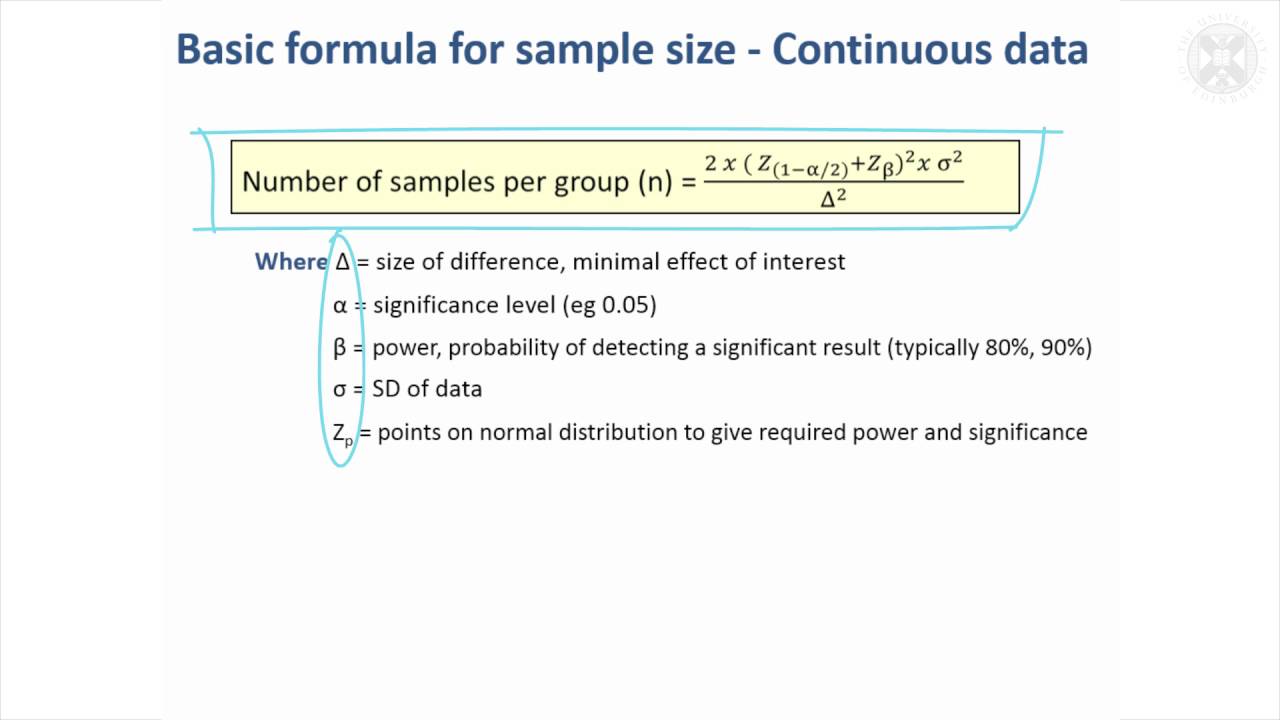
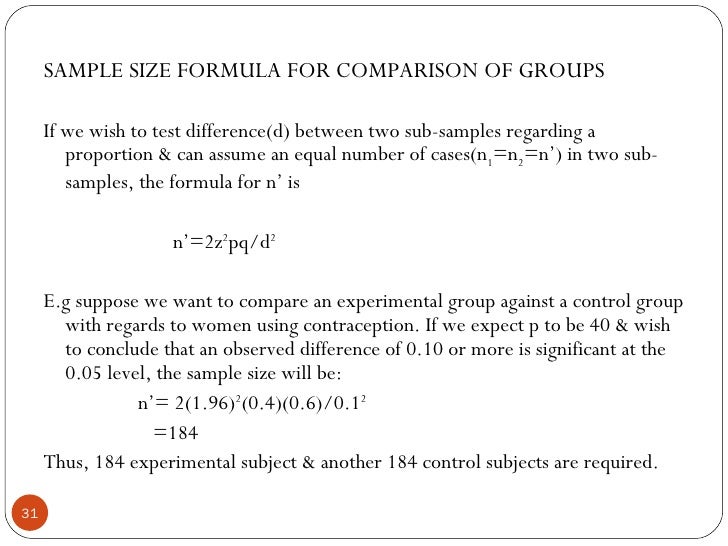
A power analysis calculates for varying sample sizes a probability power β of finding a statistically significant result at chosen type i error α for a given population effect size cohen 1988. There are certain factors to consider and there is no easy answer. Nearly all quantitative studies can be subjected to a sample size calculation.